Copyright © 1999-2006 Samuel L. Baker
Cost
Basic concepts:
-
Opportunity cost
-
Total cost
-
Fixed cost
-
Variable cost
-
Average cost
-
Marginal cost
The Economic Problem
The basic economic problem is the scarcity of social resources to
satisfy
human wants and needs.
-
An economic system must make choices about the allocation of resources
among the many possible uses.
-
The economic system also chooses how the goods and services are
distributed
-- who gets what.
Cost and the necessity of choice, even in health care
When a high percentage of all spending in our economy is for health
care, we wonder if some of the resources going into health care could
be
better used elsewhere, as
-
other kinds of health care, that might give more benefit for the same
resources
-
other kinds of health-enhancing investments besides health care, such
as
public health or health education
-
consumption goods and services that might enhance our lives more
than spending on certain kinds of health care would,
-
or as investments outside of health care that might improve our future
ability to produce
goods and services more than some investments in health might.
Opportunity Cost
Opportunity cost is the most fundamental cost concept.
The opportunity cost of doing or getting something is:
-
what you could have done or gotten instead.
Opportunity cost is what you forgo.
Example: The opportunity cost of buying a box of Cracklin Oat Bran
is one-and-a half boxes of Wheat Chex, if that's your second favorite
cereal.
Example: Your opportunity cost for taking this class includes:
-
Whatever else you could have bought with your tuition and fee money,
plus
-
the work, family participation, rest, or recreation that you are not
doing
because you are spending time doing this.
Opportunity cost is not resources used
Strictly speaking, the cost of something is not the resources used
up to get it.
Instead, the cost is what else you could have done with those
resources.
Resources have value only because you can use them to make goods and
services that have value.
Using prices for costs
Opportunity cost can be hard to use in practice.
Dollar costs (prices) are
-
easier to determine and
-
easier to add up.
Nevertheless, we should not lose sight of opportunity cost.
For example:
-
saving medical institutional costs by discharging patients early, but
-
adding opportunity costs for family members drafted into being home
caregivers
Costs and prices
Prices can reflect society's opportunity cost
-
"Reflect" here means that the ratio of prices of any two goods or
services
is the opportunity cost of the one in terms of the other.
If the market system works properly then the price ratio of any
two goods or services tells you what the social tradeoff actually is,
how
many of good X you give up to get each unit of good Y.
For this to work properly, you have to have strong competition and
savvy
consumers. Competition will then force the sellers to be efficient, and
provide goods and services at prices in line with costs.
The issue of how well the market works is one to which
we will return
in a future week, when market concepts are discussed. For an example of
what can go wrong, though, consider petroleum and ethanol. OPEC,
the oil cartel, manipulates the price of oil. The U.S. government
indirectly manipulates the price of ethanol, as does (according to some
observers) the Archer Daniels Midland agricultural products company.
With
all this manipulation going on, it would be astounding if the ratio of
gasoline and ethanol prices was anywhere close to the how much ethanol
the world would have to give up to get one more gallon of gasoline.
Even if prices don't reflect social opportunity cost,
they can reflect
an individual's opportunity cost, if the good or service is
something
you have to buy. If you have a motor you need to operate, and it
can run on gasoline or ethanol, and you cannot make your own gasoline
or
ethanol, then the opportunity cost of a gallon of gasoline in terms of
ethanol really is the the price of a gallon of gasoline divided by the
price of a gallon of ethanol.
-
That paragraph may have made the concept seem more complicated than it
really is. Suppose a gallon of gasoline costs $2.00, and a gallon
of ethanol costs $1.00. Each gallon of gasoline you buy means you
can't buy $2/$1 = 2 gallons of ethanol. The opportunity cost of a
gallon of gasoline is 2 gallons of ethanol.
That completes a chunk of material. Now
I'm about to change the subject and talk about specific money cost
concepts. This might be a good time to take a break. Use the link near
the top of this page to jump back to here when you are ready to resume.
Money cost concepts
In this section, we assume that we can use dollar costs for
costs.
We go over some concepts that are used in cost accounting.
The concepts are:
-
Total cost
-
Fixed cost
-
Variable cost
-
Marginal cost
-
Average cost
Total cost
... is a function (math concept) of quantity
Total cost = TC(Q)
Total cost at Q = the total cost per unit of time of producing Q
units
of output per unit of time
Costs are "flows," not "stocks"
The Q in the TC(Q) formula stands for Quantity per Unit of Time.
All our cost measures
including total cost, fixed cost, variable cost, marginal
cost,
average cost
have a time dimension. They are denominated in units of currency per
unit
of time.
For example, a U.S. firm presenting annual budget numbers would use
"dollars per year" as its cost units. For a monthly budget, the cost
units
would be dollars per month.
For brevity, I'll leave "per unit of time" out sometimes, but it's
always
implicitly there.
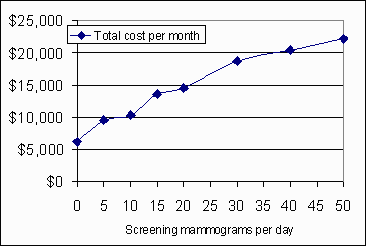
Total cost example
Here's the total cost per month of providing different numbers of
screening
mammograms per day.
Output rate
Mammograms/day |
0 |
5 |
10 |
15 |
20 |
30 |
40 |
50 |
| Total cost per month |
$6,172 |
$9,462 |
$10,337 |
$13,627 |
$14,502 |
$18,667 |
$20,417 |
$22,167 |
Source: Physician Payment Review Commission, The Costs of Providing
Screening
Mammography, 1989. This study was done just after Medicare
started
paying for screening mammograms.
The term "Total cost" is used to refer to the whole table,
representing
the relationship between output flow and cost flow. It's not just
any one number in the table, unless you say something like, "the total
cost at an output of 30 per day."
Total cost graphed
 Larger
Larger
Total cost is an increasing function of quantity. Usually, the
more your produce, the more your total cost.
Fixed cost
The cost of producing 0 output in a given time period.
Fixed cost = costs that can't be avoided in the "short run"
"Short run" means a time period in which many costs can't be
avoided.
(Wait -- isn't that circular?)
Fixed cost is a function of Q (quantity per unit of time) in the
trivial
sense that it's a constant function. Fixed cost is the same at
all
levels of Q.
Fixed cost example
First let's do the fixed cost associated with the capital outlay.
The capital outlay is a lump of money we need
before we start.
| Capital outlay required before the first patient
is seen: |
| Mammography unit and processor |
$80,000 |
| Start-up supplies |
$2,000 |
| Property improvements |
$15,000 |
| Furniture |
$5,000 |
| Office equipment |
$3,500 |
| Miscellaneous |
$500 |
| Capital outlay -- total of above |
$106,000 |
The capital outlay is a stock, really, rather than
a flow. To use our cost concepts, we have to convert it to a
flow.
We do that by imagining that we borrow the money and then pay back the
loan over a period of years at so many dollars per month. That "so many
dollars" per month is part of our fixed cost flow.
Amortized capital cost per month
at a 0.12 interest rate for 6 years.
This is the monthly fixed cost flow
associated with our initial capital outlay. |
$2,072 |
There are other fixed costs per month. These are costs that recur
monthly independent of how many patients come through.
| Other fixed costs per month |
| Maintenance |
$425 |
| Promotion |
$250 |
| Accounting |
$100 |
| Insurance |
$100 |
| Rent |
$875 |
| Telephone |
$100 |
| Taxes |
$750 |
| Clerk/Receptionist salary and benefits |
$1,500 |
| Other fixed costs -- total per month |
$4,100 |
Combine the information from the two tables to get the
total fixed cost
flow per month.
| Summary of fixed costs Sound
applet |
| Monthly capital cost |
$2,072 |
| Recurring fixed cost |
$4,100 |
| Total fixed cost flow per month |
$6,172 |
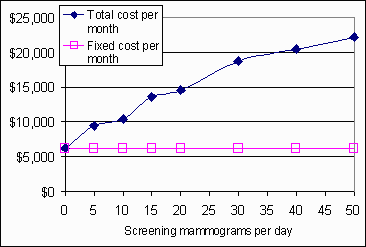
Fixed cost in the cost table
Let's add a row for fixed cost to the total cost table we saw earlier.
| Output |
0 |
5 |
10 |
15 |
20 |
30 |
40 |
50 |
| Total cost |
$6,172 |
$9,462 |
$10,337 |
$13,627 |
$14,502 |
$18,667 |
$20,417 |
$22,167 |
| Fixed cost |
$6,172 |
$6,172 |
$6,172 |
$6,172 |
$6,172 |
$6,172 |
$6,172 |
$6,172 |
The fixed cost is the same at all output rates. That is why it's called
"fixed."
The fixed cost is the total cost of being open
but producing 0.
Total Cost and Fixed Cost graphed
 Larger
Larger
Fixed cost goes straight across, because it's "fixed" -- the same -- at
all output rates.
Variable cost
Variable cost equals total cost minus fixed cost.
-
The variable cost is extra cost of producing Q, above the cost of
producing
0.
-
The variable cost is a function of Q.
In the "long run," all costs are variable. (Circular definition again!)
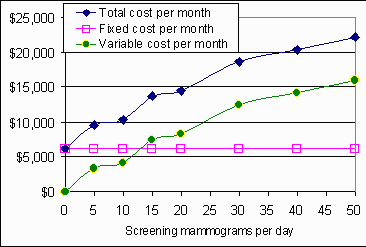
Variable cost example
Here are the variable costs for screening mammograms.
| Variable costs per month (20 working
days per
month) |
|
|
Tests per day |
| Cost category |
Unit cost |
5 |
10 |
15 |
20 |
30 |
40 |
50 |
| Radiological technologist |
|
$2,415 |
$2,415 |
$4,830 |
$4,830 |
$7,245 |
$7,245 |
$7,245 |
| Film |
$3.00 |
$300 |
$600 |
$900 |
$1,200 |
$1,800 |
$2,400 |
$3,000 |
| Medical Records |
$2.00 |
$200 |
$400 |
$600 |
$800 |
$1,200 |
$1,600 |
$2,000 |
| Supplies and miscellaneous |
$2.00 |
$200 |
$400 |
$600 |
$800 |
$1,200 |
$1,600 |
$2,000 |
| Postage |
$1.00 |
$100 |
$200 |
$300 |
$400 |
$600 |
$800 |
$1,000 |
| Forms |
$0.75 |
$75 |
$150 |
$225 |
$300 |
$450 |
$600 |
$750 |
Total monthly variable cost
(all above added up) |
|
$3,290 |
$4,165 |
$7,455 |
$8,330 |
$12,495 |
$14,245 |
$15,995 |
The costs in this table vary with the output rate, so they are variable
costs.
Variable cost graphed
 Larger
Larger
Variable cost goes up in parallel with total cost.
Marginal cost
Marginal cost is
-
Total cost at output = Q
-
minus
-
total cost at output = Q-1.
Marginal cost is the additional cost of producing one more.
-
Or the reduction in cost from producing one less.
Marginal cost example
Calculating the marginal cost for our example is a bit tricky, because
the radiological technologist is "lumpy."
-
"Lumpy" means not continuously variable.
-
The technologist is somewhat of a fixed cost over small changes in
output
rate,
-
unless you can hire a part-time technologist. There's no allowance for
that in these numbers, so let's assume that you can only hire full-time
technologists.
To start with marginal cost, let's put the technologist's costs aside
for a moment, and first do the marginal cost for the items for which we
have unit costs. Those are straightforward.
| Cost category |
Unit cost
(cost per mammogram) |
| Film |
$3.00 |
| Medical Records |
$2.00 |
| Supplies and miscellaneous |
$2.00 |
| Postage |
$1.00 |
| Forms |
$0.75 |
Adding the above shows that film, medical records, supplies and
miscellaneous,
postage, and forms cost $8.75 per mammogram.
-
(There is also the physician's fee, which was $12 per test. Let's
leave that out for now.)
$8.75 is therefore the marginal cost of a screening mammogram
if the technologist is going to be there anyway.
This means: If a woman walks in unexpectedly and offers $8.76
for a screening mammogram,
and your technologist is not busy,
then you can make $0.01 by doing a mammogram for her.
Marginal cost of larger output changes that mean adding a
technologist
If you are considering signing a contract to provide, say, 5 more
mammograms
per day,
$8.75 may not be your whole marginal cost per mammogram, because you
may have to add a technologist, depending on whether or not your
current technologists are working to capacity.
Here's our table again, with marginal cost lines added. If we
consider only these specific output rates (0, 5, 10, etc.), it
simplifies
our calculation.
Tests per day
|
0 |
5 |
10 |
15 |
20 |
30 |
40 |
50 |
| Tests per month |
0 |
100 |
200 |
300 |
400 |
600 |
800 |
1000 |
| Total cost |
$6,172 |
$9,462 |
$10,337 |
$13,627 |
$14,502 |
$18,667 |
$20,417 |
$22,167 |
| Fixed cost |
$6,172 |
$6,172 |
$6,172 |
$6,172 |
$6,172 |
$6,172 |
$6,172 |
$6,172 |
Technologists
needed
|
0 |
1 |
1 |
2 |
2 |
3 |
3 |
3 |
| Variable cost |
$0 |
$3,290 |
$4,165 |
$7,455 |
$8,330 |
$12,495 |
$14,245 |
$15,995 |
Marginal cost
from previous output level |
Not
applicable |
$3,290 |
$875 |
$3,290 |
$875 |
$4,165 |
$1,750 |
$1,750 |
Corresponding
marginal cost per unit |
Not
applicable |
$32.90 |
$8.75 |
$32.90 |
$8.75 |
$20.83 |
$8.75 |
$8.75 |
The lumpiness of the technologist makes the marginal cost jump up or
down,
depending on whether we do or do not have to add a technologist to
achieve
the next higher output rate.
The marginal cost is high when we have to add a technologist. It's
low otherwise.
Average cost and marginal cost diagram
Economies of scale
Once we grow past 30 per day, we can expand by more tens to 40 or 50
per day without having to add more technologists.
-
This is an advantage to size, an "economy of scale" in the jargon.
Marginal cost and the price we can afford to charge
Here's a small slice of that table again, for your reference.
| Tests per day |
0 |
5 |
| Tests per 20-day month |
0 |
100 |
| Total cost |
$6,172 |
$9,462 |
| Fixed cost |
$6,172 |
$6,172 |
| Technologists needed |
0 |
1 |
| Variable cost |
$0 |
$3,290 |
| Marginal cost from previous output level |
Not applicable |
$3,290 |
Corresponding marginal cost per unit
(row above divided by 100 tests per day) |
Not applicable |
$32.90 |
If we're currently doing zero business, and we have an opportunity to
contract
for 5 films a day, we must charge at least $32.90 per film to break
even
on the 5 films.
$32.90 is the marginal cost per film of going from 0 films per day
to five.
-
(Not counting the doctor's $12 fee.)
| Tests per day |
30 |
40 |
| Tests per 20-day month |
600 |
800 |
| Total cost |
$18,667 |
$20,417 |
| Fixed cost |
$6,172 |
$6,172 |
| Technologists needed |
3 |
3 |
| Variable cost |
$12,495 |
$14,245 |
| Marginal cost from previous output level |
$4,165 |
$1,750 |
| Corresponding marginal cost per unit |
$20.83 |
$8.75 |
If we're already doing 30 tests per day, we can make money if we get
any
more than $8.75 each for the extra tests above 30.
-
Again, not counting the doctor's $12 fee that we'd want to pass through
to the patient.
Marginal cost is the concept to use when considering changes.
You compare the costs with the change
to the cost without the change.
The difference is the marginal cost of the change.
Compare that with the marginal benefit of the change to decide
whether
the change is advantageous.
Average cost
Average cost is
Total cost at output = Q, divided by Q.
-
Average cost is sometimes mistakenly used in place of marginal cost.
Examples
of that come in a later lecture
Average cost can tell you one thing: Whether you're making money
overall.
-
Profit = Revenue minus cost.
-
So Profit per unit = Revenue/(Units sold) minus Average Cost.
If you charge all customers the same price
-
(in health care, you generally don't. But, suppose you did.)
then Revenue/(Units sold) = your price.
-
Revenue is the total amount you take in.
-
Revenue = Price times Quantity.
-
Therefore Price equals Revenue divided by Quantity.
Profit = Revenue minus Cost,
so profit per unit = Price minus Average Cost.
-
If Price exceeds Average Cost then your unit profit is positive.
-
If the price is less than the average cost, your average profit per
unit
is negative.
Average cost = Total cost divided by the Number of Tests = TC(Q)/Q
| Tests per day |
0 |
5 |
10 |
15 |
20 |
30 |
40 |
50 |
| Tests per month |
0 |
100 |
200 |
300 |
400 |
600 |
800 |
1000 |
| Total cost |
$6,172 |
$9,462 |
$10,337 |
$13,627 |
$14,502 |
$18,667 |
$20,417 |
$22,167 |
| Average cost |
(Can't divide
by 0.) |
$94.62 |
$51.69 |
$45.42 |
$36.26 |
$31.11 |
$25.52 |
$22.17 |
Average cost falls as tests per month goes up -- another indication of
economies of scale.
Average Cost and Marginal Cost
| Tests per day |
0 |
5 |
10 |
15 |
20 |
30 |
40 |
50 |
| Tests per month |
0 |
100 |
200 |
300 |
400 |
600 |
800 |
1000 |
| Total cost |
$6,172 |
$9,462 |
$10,337 |
$13,627 |
$14,502 |
$18,667 |
$20,417 |
$22,167 |
| Average cost |
Can't divide
by 0 |
$94.62 |
$51.69 |
$45.42 |
$36.26 |
$31.11 |
$25.52 |
$22.17 |
Marginal cost
from previous output level |
Not
applicable |
$3,290 |
$875 |
$3,290 |
$875 |
$4,165 |
$1,750 |
$1,750 |
Corresponding
marginal cost per unit |
Not
applicable |
$32.90 |
$8.75 |
$32.90 |
$8.75 |
$20.83 |
$8.75 |
$8.75 |
Look at the 50 column:
-
The marginal cost per test is $8.75
-
but the average cost is $22.17.
Can we really provide extra tests at just over $8.75 each and make
money?
-
Yes, if we don't have to give all our customers that price.
Offering a group a price just above its marginal cost will let us make
money on that group.
-
But if we offer all customers prices just above their marginal costs,
we
won't cover our fixed costs, so we'll lose money overall.
Price discrimination
-
Jargon term for charging different customers different prices.
-
Not illegal.
-
In health care, often encouraged and expected.
Average cost as the break-even price
| Tests per day |
0 |
5 |
10 |
15 |
20 |
30 |
40 |
50 |
| Tests per month |
0 |
100 |
200 |
300 |
400 |
600 |
800 |
1000 |
| Total cost |
$6,172 |
$9,462 |
$10,337 |
$13,627 |
$14,502 |
$18,667 |
$20,417 |
$22,167 |
| Average cost |
Can't divide
by 0 |
$94.62 |
$51.69 |
$45.42 |
$36.26 |
$31.11 |
$25.52 |
$22.17 |
We make money overall if our average price to all customers is greater
than the average cost.
-
In this example, if our output rate is about 50 per day, we make money
overall if our average price for all customers is at least $22.17.
Short-run and long-run decisions
In the short run, it pays to sell to any customer who'll pay marginal
cost.
-
Even if you lose money overall, you're better off than not selling.
In the long run, when you can get out of your fixed cost, you shut down
if your average price is not more than average cost.
Key cost concepts review
Opportunity cost
-
what you give up to get something
Monetary cost concepts:
-
Total cost -- the dollar value of what you give up by being in business
and operating at your current rate.
-
Fixed cost -- the dollar value of what you give up by being in
business, even if you produce nothing.
-
Variable cost -- the dollar value of what you give up
to produce at your current rate, over and above your fixed cost.
-
Marginal cost -- the dollar value of what you give up
to add one unit to your rate of production.
-
Average cost -- total cost divided by output rate.
The views and opinions expressed in this page are strictly those of the
page author. The contents of this page have not been reviewed or
approved
by the University of South Carolina.
E-mail:
 Larger
Larger
 Larger
Larger
 Larger
Larger